算法之中缀表达式和后缀表达式
一、后缀表达式求值
后缀表达式也叫逆波兰表达式,其求值过程可以用到栈来辅助存储。
假定待求值的后缀表达式为:6 5 2 3 + 8 * + 3 + *,则其求值过程如下:
(1)遍历表达式,遇到的数字首先放入栈中,依次读入6 5 2 3 此时栈如下所示:
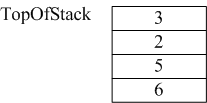
(2)接着读到“+”,则从栈中弹出3和2,执行3+2,计算结果等于5,并将5压入到栈中。
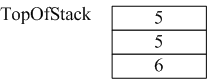
(3)然后读到8(数字入栈),将其直接放入栈中。
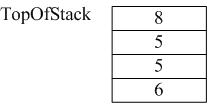
(4)读到“*”,弹出8和5,执行8*5,并将结果40压入栈中。
而后过程类似,读到“+”,将40和5弹出,将40+5的结果45压入栈...以此类推。最后求的值288。
代码:
#include<iostream>#include<stack>#include<stdio.h>#include<string.h>using namespace std;int main(){ string PostArray; int len,i,a,b; while(cin>>PostArray){ stack<int> Stack; len = PostArray.length(); for(i = 0;i < len;i++){ //跳过空格 if(PostArray[i] == ' '){ continue; } //如果是数字则入栈 if(PostArray[i] >= '0' && PostArray[i] <= '9'){ Stack.push(PostArray[i] - '0'); } //如果是字符则从栈读出两个数进行运算 else{ //算数a出栈 a = Stack.top(); Stack.pop(); //算法b出栈 b = Stack.top(); Stack.pop(); //进行运算(+ - * /) if(PostArray[i] == '+'){ Stack.push(a + b); } else if(PostArray[i] == '-'){ Stack.push(a - b); } else if(PostArray[i] == '*'){ Stack.push(a * b); } else if(PostArray[i] == '/'){ Stack.push(a / b); } } }//for printf("%d\n",Stack.top()); }//while return 0;}